James Franklin
Mathematics has always been a core part of western education, from the medieval quadrivium to the large amount of arithmetic and algebra still compulsory in high schools. It is an essential part. Its commitment to exactitude and to rigid demonstration balances humanist subjects devoted to appreciation and rhetoric as well as giving the lie to postmodernist insinuations that all “truths” are subject to political negotiation.
The ancients and deductive proof
In the mid-fifth century BC, the Parthenon was rising over Athens, built according to the best geometrical principles. The construction lines are not visible on it, though on some other Greek temples they are. Among the tragedians, artists, sophists and merchants creating Western civilisation in the city below, a number of visitors from out of town brought an interest in what we would now call scientific questions. According to Parmenides of Elea, for example, thinking about the geometry of eclipses is enough for a convincing argument that the earth is round, even for someone fixed in one place on the earth’s surface. Others, including Plato’s associates Eudoxus and Theaetetus, realised that there was something special about pure geometry: it could be organised as a vast deductive structure in which the less obvious theorems were deduced by small and obvious steps from a small number of initial obviously true axioms. Thus a complicated and non-obvious truth, like Pythagoras’s theorem that in any right-angled triangle the square on the hypotenuse (c in the diagram) has area equal to the sum of the squares on the other two sides (a and b), could be established with certainty by following it back to self-evident axioms about space.
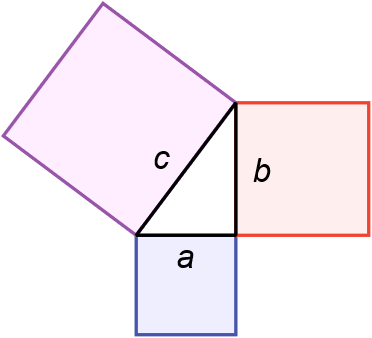
Pythagoras’s Theorem (Image: Wikipedia commons)
The Greeks immediately realised there was something special about deductive proof as a way of acquiring knowledge. Proof somehow gets to a deeper level of reality. It reveals not only what is so, but what must be so. And the proof allows us not only to know what must be so, but to understand why it must be so.
The deductive structure of mathematics was laid out in the thirteen books of Euclid’s Elements and in many thousands of mathematical books and papers since. The method of deductive proof generates indefinitely many results. It is just a matter of human interest and energy keeping up with them.
The certainty of basic mathematical truths
A very simple example shows how the initial and most basic results of mathematics are known with certainty.
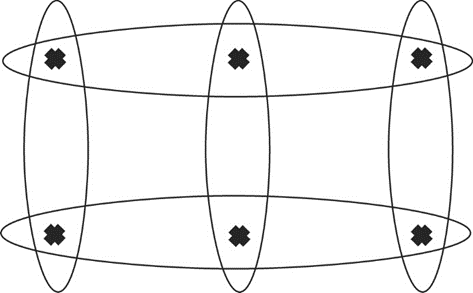
Why 2 × 3 = 3 × 2
In the figure, the six crosses are arranged both as two rows of 3 and as three columns of 2. Since they are the same six crosses, 2 × 3 = 3 × 2. We can literally see not only that 2 × 3 = 3 × 2, but that 2 × 3 must be 3 × 2.
Further, we can easily see that the same reasoning applies if we add more rows and columns. If we have m columns and n rows, the same reasoning is valid. Therefore m × n = n × m, for any numbers m and n. So the insight produces an infinite number of truths, all of them understood to be true with certainty. These truths apply directly to the real world of actual crosses written on paper, or of any other objects whatsoever. There is no need for getting out in the wet and observing, but the abstract truths predict what will be observed by anyone who does get out there.
Excited by these possibilities, Aristotle proposed in his Posterior Analytics that all sciences should follow the model of mathematics, with immediately understood axioms supporting a superstructure of more complex theorems, and proofs explaining why the theorems were true. That did not quite work out. Contrary to Aristotle’s hope, in the natural and human sciences observation and measurement are still essential to establishing the truth of theories. Only in mathematics, and in some closely related fields like computer science and just possibly ethics, is proof in the full sense feasible. Thus mathematics remains the ideal training ground for the human faculty of understanding and proof.
Mathematics at the centre of western education
Western education has not lost sight of the point of mathematics and has always made it central to education, despite the fact that it is quite hard to learn and subject to a certain degree of customer resistance.
Medieval liberal tertiary education, the preparation for specialised studies such as theology, law and medicine, was divided into two levels, the trivium and the quadrivium. The trivium consisted of grammar, logic and rhetoric, that is, studies in words and how they work. Then came the quadrivium: arithmetic, geometry, music (theory) and astronomy, all of which are mathematics in one form or another. Soon the Italian merchant schools of the later middle ages made the remarkable discovery that calculation with indefinitely large numbers could be reduced to rules and taught to seven-year-olds. Basic numeracy became widespread along with basic literacy. The new technologies of early modern times found a population able to understand and manipulate them.
There was some backsliding from the humanists of the Renaissance, who preferred words, but the Scientific Revolution of the seventeenth century was and was seen to be based on mathematics and data instead of wordy disputation. Galileo explains:
If what we are discussing were a point of law or of the humanities, in which neither true nor false exists, one might trust in subtlety of mind and readiness of tongue and in the greater experience of the writers, and expect him who excelled in those things to make his reasoning more plausible, and one might judge it to be the best. But in natural sciences whose conclusions are true and necessary and have nothing to do with human will, one must take care not to place oneself in the defense of error; for here a thousand Demostheneses and a thousand Aristotles would be left in the lurch by every mediocre wit who happened to hit upon the truth for himself.
In Victorian England, the study of Euclid was presumed suitable for training boys of the upper classes in the intellectual tasks that awaited them such as governing India.
The upshot of this long process was that mathematics – and mathematics at a substantially high level – has become a compulsory part of education across the board. That has applied not just in Western countries. The Soviet Union, for all its Marxist ideology in the humanities, left pure mathematics alone and maintained a very high standard of mathematics education in schools. East Asian countries have surpassed Western ones in school mathematics education, having successfully grafted Western mathematics onto their cultural traditions. The culture of research mathematics is the same worldwide, with the same symbols used everywhere and virtually everything written in English. Mathematics is a universal culture – as international and standardised as air traffic control but impacting vastly more people.
In recent decades, the character of mathematics has changed – or rather broadened: it has become the enabling science behind the complexity of contemporary knowledge, from gene interpretation to bank risk. Mathematical understanding is all the more necessary for future jobs, as well as remaining, as ever, a prophylactic against the more corrosive philosophical views emanating from the humanities.







